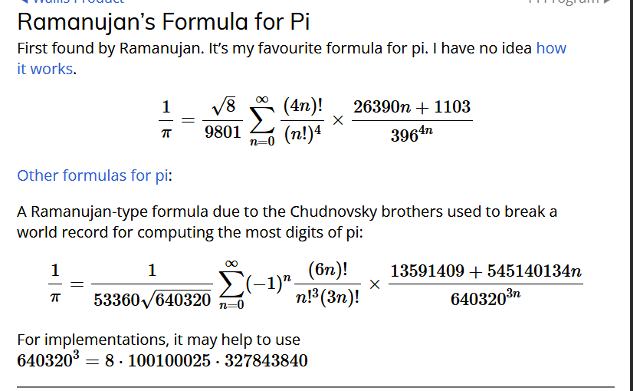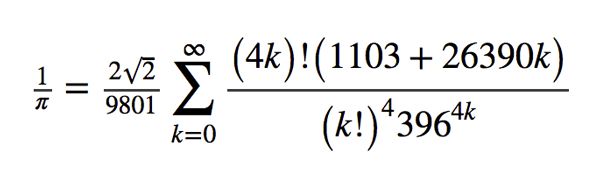
Joseph T Noony on Twitter: "Ramanujan's formula and its variants are today used by supercomputer algorithms for calculating pi correct to millions of decimals of accuracy! What a true genius he was

0027: Part 6, Ramanujan's pi formulas and the hypergeometric function - A Collection of Algebraic Identities

Ramanujan-like formulae for $$\pi $$ and $$1/\pi $$ via Gould–Hsu inverse series relations | SpringerLink
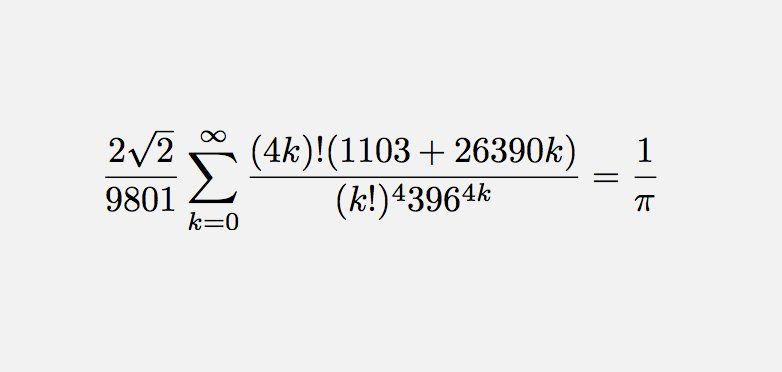
Fermat's Library on Twitter: "Ramanujan discovered this peculiar way to represent 1/π. https://t.co/nyge5IeqFM" / Twitter

𝐒𝐫𝐢𝐧𝐢𝐯𝐚𝐬𝐚 𝐑𝐚𝐠𝐡𝐚𝐯𝐚 ζ(1/2 + i σₙ )=0 on Twitter: "In the year 1914, Srinivasa Ramanujan published a paper titled 'Modular Equations & Approximations to Pi' in Cambridge journal. In that Ramanujan gave

0027: Part 6, Ramanujan's pi formulas and the hypergeometric function - A Collection of Algebraic Identities

python 3.x - Estimating value of 1/pi using Ramajunam equation, returning wrong value when comparing with (1/math.pi) - Stack Overflow






![Happy Pi Day 2020! The Srinivasa Ramanujan Series | Python [ITA] - YouTube Happy Pi Day 2020! The Srinivasa Ramanujan Series | Python [ITA] - YouTube](https://i.ytimg.com/vi/ri7twYfBckQ/hqdefault.jpg)